Invert a Binary Tree

Asked in: Google, Amazon
Difficulty: Medium
Understanding the problem
Problem description: Invert a binary tree.
An inverted form of a Binary Tree is another Binary Tree with left and right children of all non-leaf nodes interchanged. You may also call it the mirror of the input tree.
NOTE: The tree is binary so there could be a maximum of two child nodes. This problem was originally inspired by this tweet by Max Howell .
Now, figure out that what does the interviewer mean when he says invert a binary tree, it would be helpful when you start looking at an example.
Example 1
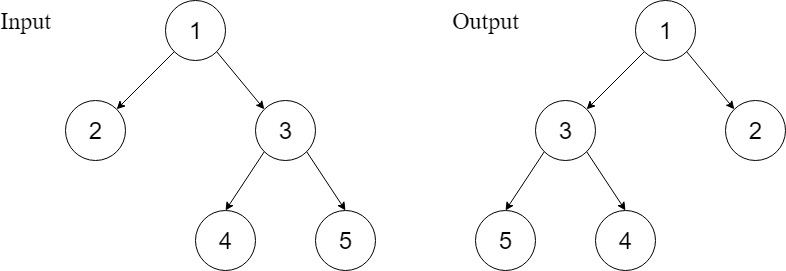
Example 2
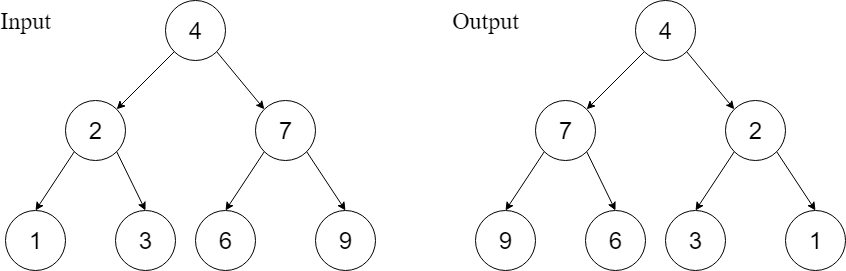
Explanation : You can observe that the root’s left pointer started pointing towards the right child and the right pointer towards the left child and a similar condition is noticed for all the sub root nodes.
The node structure for the BST passed to your function will be
class TreeNode
{
int val
TreeNode left
TreeNode right
}Recursive and iterative solutions
We will be discussing three approaches to solve this problem
- Recursive solution (similar to pre-order traversal) : Solve the problem by swapping the left and right child for the root of each subtree recursively.
- Using Iterative Preorder Traversal : We can convert the above recursive solution to iterative using stack.
- Using Level order traversal : iterative approach using queue
1. Recursive Solution
The key insight here is to realize that in order to invert a binary tree we only need to swap the children and recursively solve the two smaller sub-problems (same problem but for smaller input size) of left and right sub-tree. This looks similar to the idea of pre-order traversal.
The steps to be followed are :
- When the tree is empty return NULL . This is also our base case to stop recursive calls.
- For every node encountered we swap its left and right child before recursively inverting its left and right subtree.
Pseudo Code
// Function to invert given binary Tree using preorder traversal
void invertBinaryTree(TreeNode root)
{
// base case: if tree is empty
if (root == Null)
return
// swap left subtree with right subtree
swap(root.left, root.right)
// invert left subtree
invertBinaryTree(root.left)
// invert right subtree
invertBinaryTree(root.right)
}Complexity Analysis
In the above approach, we are traversing each node of the tree only once. Time complexity : O(n)
Space Complexity of this algorithm is proportional to the maximum depth of recursion tree generated which is equal to the height of the tree (h).
Space complexity : O(h) for recursion call stack, where h is the height of the tree.
Critical ideas to think
- Can we use idea similar to post-order traversal for solving this problem? or, can we use some other recursive idea for solution?
- Why recursion help us to solve the tree problems easily? Explore the properties of Pre-order, In-Order and Post-Order traversal.
- What is the space complexity in the worst and best-case scenario?
2. Using Iterative Preorder Traversal
Here, a Iterative Preorder Traversal is used to traverse the tree using a LIFO stack. To convert recursive procedures to iterative, we need an explicit stack during the implementation.
Solution Steps
- When the tree is empty return NULL.
- Create an empty stack S and push root node to stack.
- Do following while S is not empty :
- Pop an item from stack S and swap the left child with right child
- Push right child of popped item to the stack S.
- Push left child of popped item to the stack S
Right child is pushed before left child to make sure that left subtree is processed first.
Pseudo Code
// Iterative Function to invert given binary Tree using stack
void invertBinaryTree(TreeNode root)
{
// base case: if tree is empty
if (root is null)
return
// create an empty stack and push root node
stack S
S.push(root)
// iterate until the stack is not empty
while (S is not empty)
{
// pop top node from stack
TreeNode curr = S.top()
S.pop()
// swap left child with right child
swap(curr.left, curr.right)
// push right child of popped node to the stack
if (curr.right)
S.push(curr.right)
// push left child of popped node to the stack
if (curr.left)
S.push(curr.left)
}
}Complexity Analysis
For each node in the tree, we are performing push() and pop() operation only once. Total no of stack operations = 2n (Think!)
Time complexity: O(n), where n is the total number of nodes.
Space Complexity: O(n) (Think!)
Critical Ideas to think
- Visualize the stack operations via simple examples.
- Explore the best and worst case scenario of space complexity.
- Design iterative algorithms for in-order and post-order traversal (using stack).
- Can we solve this problem using level-order traversal?
3. Using Level order traversal
We can solve this problem using Level Order Traversal. Here we the traverse the tree using a FIFO Queue.
In each iteration :
- We are deleting one node from the queue : TreeNode curr = Q.dequeue()
- Swapping the left and right child : swap(curr.left, curr.right)
- Inserting left and right child to the queue
if (curr.left)
Q.enqueue(curr.left)
if (curr.right)
Q.enqueue(curr.right)Pseudo Code
// Iterative Function to invert given binary Tree using queue
void invertBinaryTree(TreeNode root)
{
// base case: if tree is empty
if (root is NULL)
return
// maintain a queue and push push root node
queue Q
Q.enqueue(root)
// iterate untill queue is not empty
while (Q is not empty)
{
// pop top node from queue
TreeNode curr = Q.dequeue()
// swap left child with right child
swap(curr.left, curr.right)
// push left child of popped node to the queue
if (curr.left)
Q.enqueue(curr.left)
// push right child of popped node to the queue
if (curr.right)
Q.enqueue(curr.right)
}
}Complexity Analysis
For each node in the tree, we are performing enqueue() and dequeue() operation only once. Total no of queue operations = 2n (Think!)
Time complexity: O(n), where n is the total number of nodes.
Space Complexity: O(n) (Think!)
Critical Ideas to think
- Can you use a similar approach if the tree is generic?
- Explore the best and worst case scenario of space complexity.
- Can we write a recursive code for level order traversal? If yes then, compare its performance with Iterative Implementation (level order traversal using queue).
Comparison of different solutions
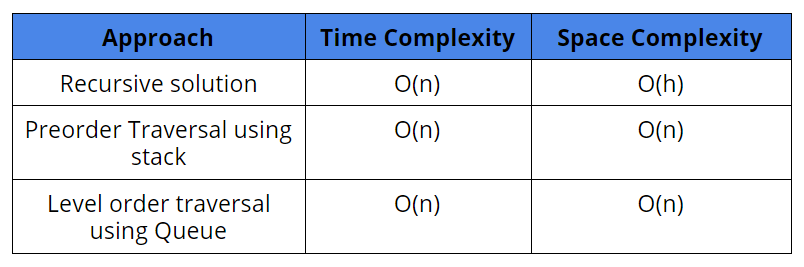
Suggested Problem to solve
- Convert a given Binary tree to a tree that holds Logical AND property
- Create a mirror tree from the given binary tree
- Find mirror of a given node in a binary tree
- Convert binary tree to threaded binary tree
- Check if two binary trees are equal or not
- Convert a given tree to its Sum Tree
If you have any more approaches or you find an error/bug in the above solutions, please comment down below.
Happy Coding! Enjoy Algorithms!
AfterAcademy Data Structure And Algorithms Online Course — Admissions Open